Gerrymandering

How it works

Gerrymandering is the process of drawing electoral districts with the goal of maximizing the number of districts where a majority of voters favor a certain political party. Gerrymandering can lead to a scenario in which a majority of districts elect representatives from a certain political party even though a majority of voters favor the other party.

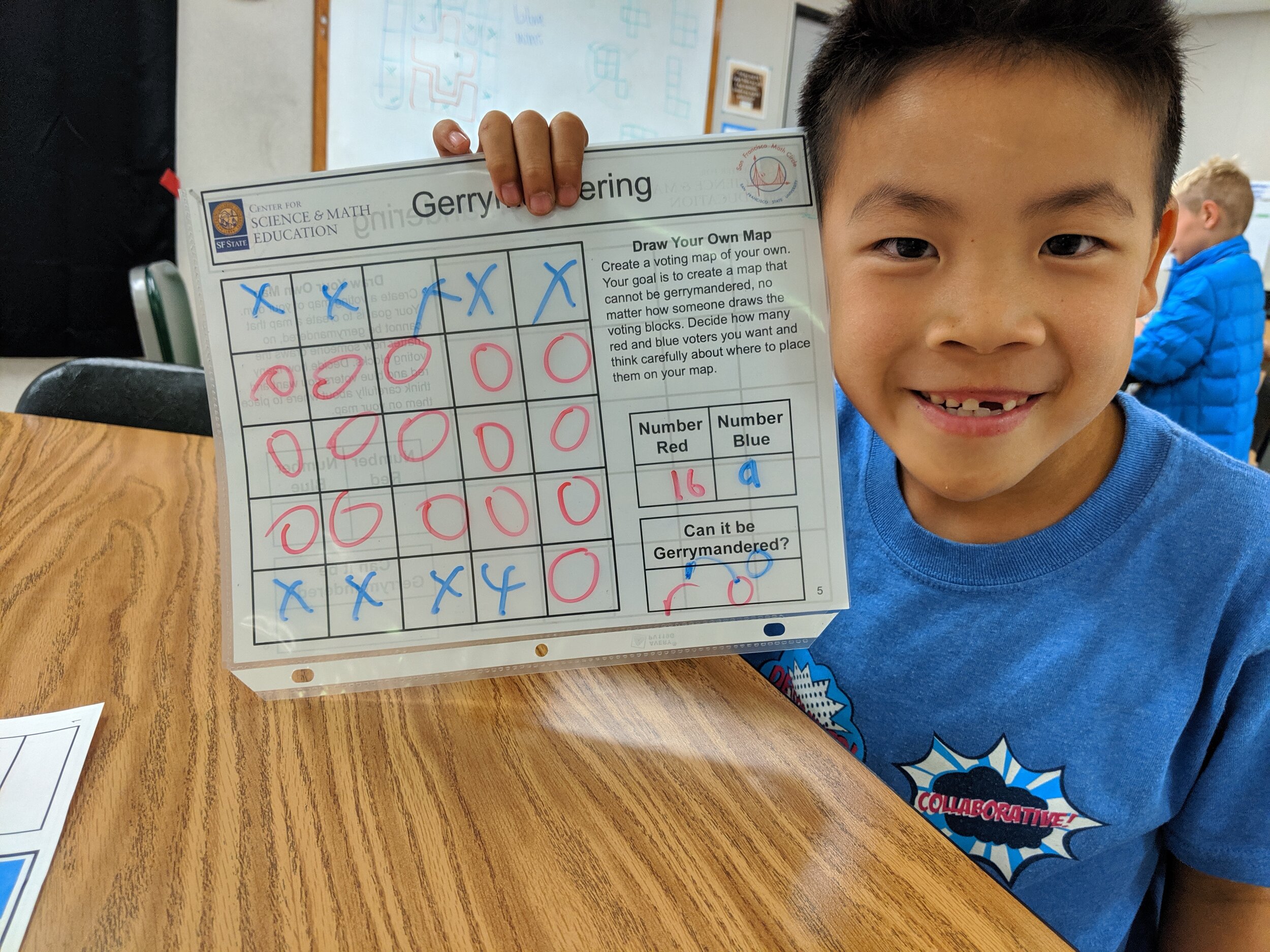
Given a map —a grid with squares colored either red or blue (with more red squares than blue, let’s say) — can you figure out how to gerrymander it so that a majority of districts have a majority of blue squares?

Are there maps that are gerrymander-proof? Is it possible to find two maps with the same number of blue squares where one can be gerrymandered and the other can’t?

Stacking the Vote handout
Red vs. Blue maps
Draw Your Own challenge
Gerrymandering challenge

Why we like this activity:

• It’s fun! The maps are visually appealing and drawing the districts is a compelling challenge.

• It helps to develop spatial reasoning.

• It connects mathematical thinking with real-world issues.

• It requires students to engage in mathematical habits of mind:

  • Finding and using strategies to gerrymander different maps.

  • Understanding and explaining why certain maps are gerrymander-proof.

  • Finding and using strategies to design gerrymander-proof maps.

• It has a low floor and high ceiling: Students can get started drawing districts by trial and error, but there is strategy to discover and deep questions to explore.

To find out more about our approach to math and math education, click here.